Unraveling the Beauty of Simple Harmonic Motion

Simple Harmonic Motion (SHM) is a fundamental concept in physics that governs the behavior of countless natural phenomena, from the oscillation of a pendulum to the vibrations of guitar strings. It is a recurring pattern of motion that follows a predictable and elegant mathematical description. In this article, we will embark on a journey to explore the captivating world of Simple Harmonic Motion, its characteristics, applications, and the profound insights it offers into the mechanics of the universe.

Understanding Simple Harmonic Motion

Simple Harmonic Motion is defined as a type of periodic motion in which an object moves back and forth around a central point, following a specific pattern. Several key characteristics define SHM:

1. Periodicity: SHM is periodic, which means that it repeats itself at regular intervals of time. The time taken for one complete cycle is called the period (T).
2. Amplitude (A): The maximum distance an object reaches from its equilibrium position during SHM.
3. Equilibrium Position: The central point around which the object oscillates without experiencing any net force.
4. Restoring Force: SHM occurs when a restoring force proportional to the displacement of the object from its equilibrium position acts on it. This force always points toward the equilibrium position, acting to bring the object back.
5. Frequency (f): The number of cycles or oscillations completed per unit of time. It is the reciprocal of the period (f = 1/T) and is measured in hertz (Hz).

Mathematical Representation

The motion of an object undergoing Simple Harmonic Motion can be described mathematically using a sinusoidal function, often a sine or cosine wave. The general equation for SHM is:

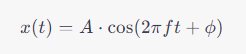
Where:

• $x(t)$ is the displacement of the object from its equilibrium position at time $t$.
• $A$ is the amplitude of the motion.
• $f$ is the frequency of the motion.
• $\varphi$ is the phase angle, which determines the initial position of the object in its oscillatory cycle.

Applications of Simple Harmonic Motion

Simple Harmonic Motion finds application in numerous aspects of science and technology:

1. Pendulum Clocks: The motion of a pendulum is a classic example of SHM and has been used for centuries in clock mechanisms to keep precise time.
2. Mechanical Systems: SHM principles are used in designing shock absorbers, springs, and damping systems in vehicles to ensure a smoother and safer ride.
3. Music: Musical instruments, such as stringed instruments like guitars and violins, produce sound through strings undergoing SHM.
4. Seismology: SHM is crucial in the study of seismic waves and helps scientists understand and predict earthquakes.
5. Optics: Laser beams and the vibrations of mirrors in optical systems are controlled using SHM for precise measurements and experiments.
6. Electronics: Oscillators, which generate stable frequencies, are essential components in electronic circuits and are used in various applications like signal generation and communication.

Conclusion

Simple Harmonic Motion is not just a theoretical concept; it is a fundamental aspect of our physical world. Its predictable and elegant nature allows us to comprehend and manipulate complex mechanical systems, making it a cornerstone of physics and engineering. Whether it’s the gentle swing of a pendulum, the vibrations of a musical instrument, or the mechanics of a shock absorber, SHM offers us a window into the beautifully rhythmic and harmonious dance of the universe.